This comprehensive overview delves into the intricate realm of reading concentration ratio, presenting a clear understanding of weight/weight (w/w) and weight/volume (w/v) units. By offering an analogy that juxtaposes the additive nature of mass with the nuanced behavior of liquids, it illuminates how combining equal volumes of liquids may yield unexpected results due to density disparities. The discourse delves into concentration units—percentages, parts per million (ppm), and parts per billion (ppb)—their conversions, and the pragmatic selection of the appropriate unit for different scenarios. We also go into into the methodology behind dilution calculations to achieve target concentrations, elucidating the interplay between product and dilution concentrations. In sum, this article will empower you to adeptly navigate the intricacies of concentration and dilution processes.
How to Express Concentration Ratios

The concentration ratio of a solution can be measured using weight/weight (w/w) and weight/volume (w/v) units. We provide an analogy to help you understand why combining equal volumes of two liquids might not always give you the sum of their volumes.
Concentration Ratio: Weight/Weight (w/w%)
In this context, it’s discussing a salt solution. For example, if you have a 1% salt solution, it means that in every 100 grams of the solution, there is 1 gram of salt. The formula used to calculate this concentration is:
%w/w = (a / a + b) * 100
Where:
“a” is the weight of the solute (salt, in this case), and
“b” is the weight of the solvent (water, in this case).
So, if you have 1 gram of salt (a) and 99 grams of water (b), the concentration would be:
%w/w = (1 / (1 + 99)) * 100 = 1%.
Concentration Ratio: Weight/Volume (w/v%)
This is another way to express concentration, but this time in terms of weight per volume. It is commonly used when a solid solute is dissolved in a liquid solvent. For example, if you have a 1% w/v salt solution, it means that in every 100 milliliters of the solution, there is 1 gram of salt. The formula used to calculate this concentration is:
%w/v = (a / c) * 100
Where:
“a” is the weight of the solute (salt, again), and
“c” is the volume of the solvent (water, in this case).
If you have 1 gram of salt (a) and you add enough water to make the total volume 100 milliliters (c), the concentration would be:
%w/v = (1 / 100) * 100 = 1%.
Analogy for Volume Mixing
Adding 1 kilogram of a substance to another 1 kilogram of the same substance will always give you a total of 2 kilograms. This is because mass is additive.
However, when dealing with liquids, adding 1 liter of one liquid to 1 liter of another liquid might not necessarily give you a total of 2 liters. This is because the two liquids can have different densities or might not mix perfectly, leading to a volume that is different from the simple sum of the initial volumes.
This text is discussing the concept of basic dilution, the process of mixing two substances (liquid A and liquid B) to create a solution. It highlights a distinction between the final weight and final volume when diluting substances and provides an example using salt and water to explain the concept.
Final Weight vs. Final Volume
Final Weight of Liquid A Mixed with B
When you mix two substances (liquid A and liquid B) together, the final weight of the mixture will be equal to the sum of the weights of the individual substances. In other words, the mass is additive.
Final Volume of Liquid A Mixed with B
However, when you mix two liquids (liquid A and liquid B), the final volume of the mixture may not necessarily be equal to the sum of the volumes of the individual liquids. This is because liquids can have different densities, resulting in a final volume that is different from the simple sum of the initial volumes.
Solute and Solvent
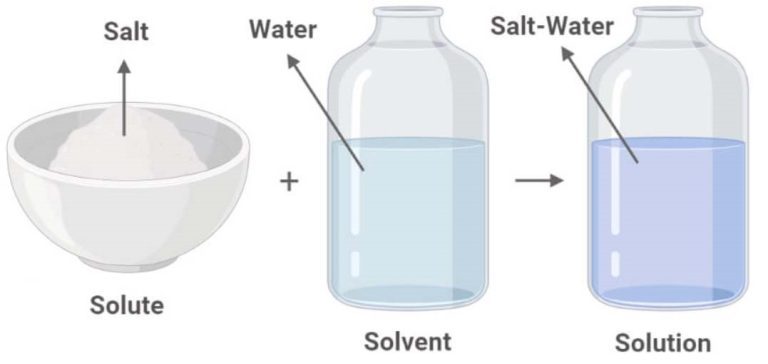
Solute (a) + Solvent (b) → Solution: This equation represents the process of creating a solution by mixing a solute (in this case, salt) with a solvent (in this case, water).
Salt + Water → Salt Solution: This is an example of the general process, where salt is dissolved in water to create a salt solution.
Concentration Units

Ratio (%)
This refers to expressing a concentration as a ratio of two quantities, usually measured in the same unit (e.g., grams to grams, liters to liters). The concentration is represented as “a/b x 100,” where “a” is the amount of the substance you’re interested in, and “b” is the total amount of the mixture.
% = a/b * 100
PPM (Parts Per Million) Conversion
Ppm is a unit used to express very small concentrations. It is calculated by multiplying the ratio “a/b” by 1,000,000. Ppm is often used to indicate the proportion of one substance in a million parts of a mixture.
PPM = a% * 1,000,000 = a * 10,000
Ppb (Parts Per Billion) Conversion
Ppb is an even smaller unit than ppm. It is calculated by multiplying the ratio “a/b” by 1,000,000,000. Ppb indicates the proportion of one substance in a billion parts of a mixture.
PPB = a% * 1,000,000,000 = a * 10,000,000
Selecting the Right Unit for Concentration Ratios

The choice of measurement unit depends on the specific application and dosage requirements. Factors like whether the dosage is high or low, and whether the dilution is being done manually or with a dilution machine, play a role in determining the appropriate unit to use.
Assumption: Volume Addition
We assume that adding volumes “a” and “b” will result in a combined volume of “a + b.” This assumption is used to illustrate the basic concept of combining volumes.
Using Ratios for Volume Measurement
When it’s not possible to measure or weigh substances by volume accurately, we suggest using ratios. Ratios provide a simpler way to express proportions when direct measurements are challenging.
Using Percentage for Dilution
When using a dilution machine that deals with concentration and dilution in percentage, it’s more convenient to use percentages as the measurement unit. This is particularly useful for cases where you’re working with larger concentrations.
Converting to Ppm
To convert a concentration from percentage to ppm, multiply by 10,000. This conversion is helpful when dealing with very small percentages or when using a dilution machine that works with ppm.
Product Concentration and Ppm
Different product concentrations can be expressed in parts per million (ppm) and percentages. For instance, a product with a concentration of 200 ppm can be expressed as 0.02% (200 divided by 10,000).
Choosing % or Ppm
When dealing with very small percentages (e.g., 0.0001%) or using a dilution machine that operates in ppm, it might be more practical to use ppm. This is because saying “500 ppm” is easier than saying “0.05%.” Compare this to the ease of saying “100g” or “0.1kg” for the same amount of substance, illustrating that the choice of unit depends on practicality and ease of communication.
Application and Dilution Method
Whether to use % or ppm depends on the specific application and how the user is performing dilutions. If dilutions are done using machines or manually, it can influence the choice of unit.
Dilution Process
Dilution involves making a solution less concentrated by adding more water or solvent For example, if you dilute with water to a concentration of 0.25%, it means that 0.25% of the total solution is the product, and the rest is water.
Calculating Dilution for Target Concentration
To dilute a product to a specific target concentration, you can use the formula: x dilution concentration = [target]. Here, represents the product concentration, [target] is the desired target concentration, and dilution concentration is the ratio of the target concentration to the product concentration.
Calculating Dilution for End Use
When determining how much to dilute a product for a particular application, you can use the formula: x dilution required = [target]. The dilution required is the ratio of the target concentration to the product concentration. This formula helps you determine the amount of product and diluent needed to achieve the desired concentration.
Dilution Calculation
The formula for calculating the dilution required to achieve a target concentration is given by:
(Product Concentration) × (Dilution Factor) = (Target Concentration)
Where:
(Product Concentration) = Concentration of the product (by default, product% is 100% = 1)
(Target Concentration) = Desired target concentration
Dilution Factor Calculation:
Dilution Factor (Dilution Concentration) = (Target Concentration) / (Product Concentration)
For example, to determine the solution concentration required for a product with a 5% target concentration of 0.5%:
Dilution Factor = (0.5% / 0.05) = 10
Example Question

Which dilution plonge to use to achieve 25ml 30L for 4772 Capture?
Step 1: Check Target Concentration
Step 1 involves checking the target concentration.
Step 2: Conversion to Percentage
Convert the given ratio to a percentage if it’s not already in percentage form.
Given: 25 ml / 20 L
Convert 25 ml to equivalent liters: 25 ml / 1000 = 0.025 L
Then, the ratio becomes: 0.025 L / 20 L = 0.00125
To express in percentage form, multiply by 100:
0.00125 * 100 = 0.125%
Therefore, 25 ml in 20 L is equivalent to 0.125%.
Step 3: Calculate Dilution Required
Calculate the dilution required to achieve the target concentration.
Dilution Required (Dilution Factor) = [Target Concentration] / [Product Concentration]
Given: Target Concentration = 0.125%, Product Concentration = 100% (or 1 as a decimal)
Dilution Factor = 0.125% / 100% = 0.00125
Therefore, the dilution required is 0.125%, which is equivalent to 0.125%.
ORAPI RECOMMENDS:
Odourless Dishwashing Liquid
Conclusion: How to Read Concentration Ratio
In conclusion, the process of measuring and understanding the concentration of solutions involves various units such as weight/weight (w/w) and weight/volume (w/v), each with its distinct formula and application. The analogy of volume mixing emphasizes that unlike mass, combining equal volumes of liquids may not always result in a straightforward sum due to density variations or imperfect mixing. We highlight the significance of different concentration units like percentages, parts per million (ppm), and parts per billion (ppb) and offer insights into choosing appropriate units based on practicality and ease of communication. Furthermore, it elucidates dilution calculations for achieving target concentrations, showcasing the interplay between product and dilution concentrations in the process. This comprehensive overview will equip you with the tools to navigate the intricacies of concentration and dilution processes effectively.